Exploring the Topology of an Annular Region

Let $X$ = ℝ² be endowed with the Euclidean topology. Consider the set:

$A = \{(x,y) \in \mathbb{R}^2 \mid 1 < x^2 + y^2 < 4\}$

which represents an open annular region in the plane (the region between two concentric circles of radii 1 and 2, excluding the boundaries).
Question: Is the Open Annular Region an Open or Closed Set?

Step 1: Checking if A is Open

A set is open in the Euclidean topology if for every point in the set, there exists an open ball around that point that is entirely contained within the set.

Let $p = (x_0, y_0)$ be a point in $A$ , meaning:

$1 < x_0^2 + y_0^2 < 4.$

We define an open ball $B_r(p)$ centered at $p$ with radius $r$ :

$B_r(p) = \{(x,y) \in \mathbb{R}^2 \mid (x - x_0)^2 + (y - y_0)^2 < r^2 \}.$

Since $p$ is strictly inside the annular region, we can always find a small enough $r$ such that $B_r(p) \subset A$ . This confirms that $A$ is an open set.

Step 2: Checking if A is Closed

A set is closed if it contains all of its limit points, meaning it contains its boundary.

The boundary of $A$ is formed by the two circles:

$\partial A = \{(x,y) \in \mathbb{R}^2 \mid x^2 + y^2 = 1 \text{ or } x^2 + y^2 = 4\}.$

These points are not included in $A$ , since $A$ consists only of points where $1 < x^2 + y^2 < 4$ . Therefore, there exist limit points (points on the boundary) that are not in $A$ , meaning that $A$ is not closed.

Step 3: Finding the Boundary of A

The boundary of a set $A$ consists of all points where every open ball centered at them contains both points from $A$ and points from $A^c$ (the complement of $A$ ).

If we take a point on the inner circle $x^2 + y^2 = 1$ , any open ball centered at that point will contain points from $A$ (where $x^2 + y^2 > 1$ ) and points from outside $A$ (where $x^2 + y^2 < 1$ ). The same occurs for the outer circle $x^2 + y^2 = 4$ .

Thus, the boundary is:

$\partial A = \{(x,y) \in \mathbb{R}^2 \mid x^2 + y^2 = 1 \text{ or } x^2 + y^2 = 4\}.$

Step 4: Modifying the Set

Now, consider a modified set $A'$ defined as:

$A' = \{(x,y) \in \mathbb{R}^2 \mid 1 \leq x^2 + y^2 \leq 4\}$

How does this change the properties of the set?

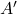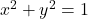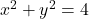$A'$ is no longer open because it includes its boundary, meaning points on the circles $x^2 + y^2 = 1$ and $x^2 + y^2 = 4$ do not have open neighborhoods fully contained in $A'$ .
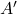$A'$ is now closed because it contains all of its limit points (the entire boundary is included).

Conclusion

Set	Open	Closed
$A$ (Open Annulus)	✅ Yes	❌ No
$A'$ (Closed Annulus)	❌ No	✅ Yes

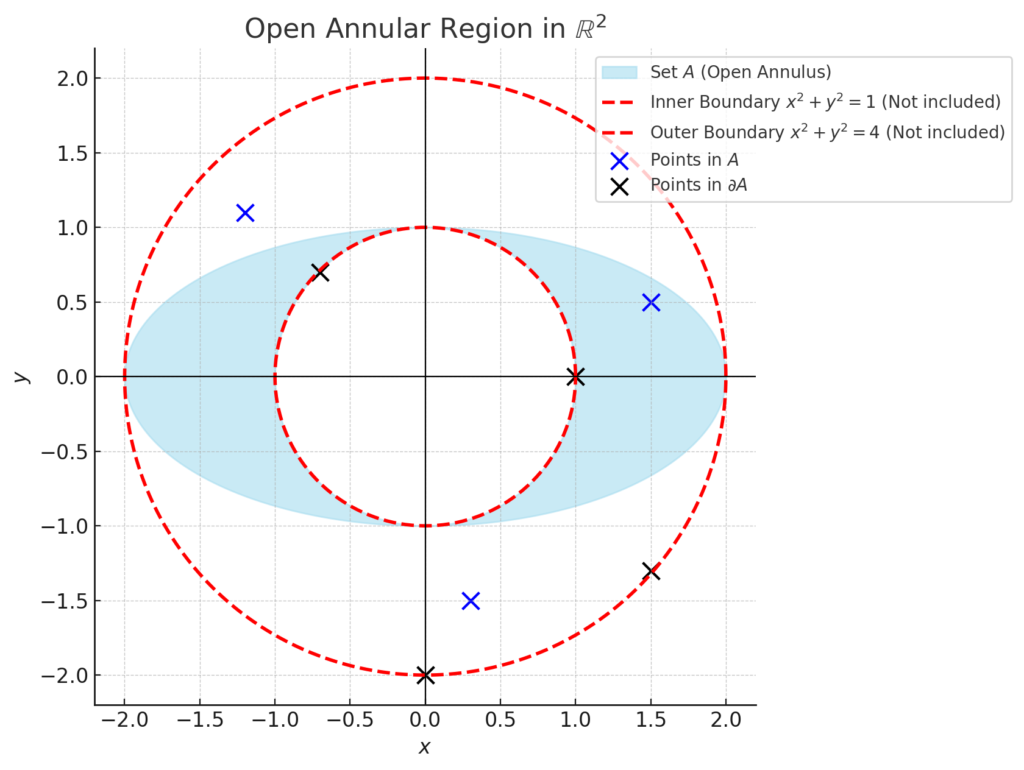
This exercise illustrates how modifying a set by including or excluding its boundary affects its openness and closedness in the Euclidean topology.

Step 1: Checking if A is Open

Step 2: Checking if A is Closed

Step 3: Finding the Boundary of A

Step 4: Modifying the Set

Conclusion

Reader Interactions